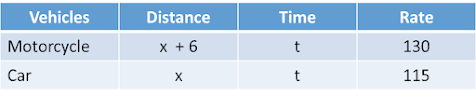
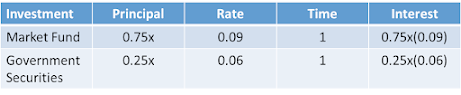
Lesson 5 – Applications of Linear Equations
Sa nakaraang mga aralin ay napag-aralan natin kunin ang solution set ng ating simultaneous equations o linear equations sa pamamagitan ng pag-plot ng graph ng mga ito, gamit ang substitution method, at elimination method. Sa leksyong ito, i-aaplay natin ang ating natutunang konsepto at pamamaraan sa paglutas ng mga word problems na kinasasangkutan ng linear equations.
Ang kaalaman kung paano lutasin ang mga linear na equation ay maaaring gamitin sa mga sumusunod: number problems, geometric problems, work problems, uniform motion problems, investment problems, at mixture problems na maaaring maging kapaki-pakinabang sa ating pang-araw-araw na buhay. Talakayin natin ang bawat uri ng word problem nang detalyado. Uunahin natin ang mga word problems na may kinalaman sa number, geometric figures, at work problems.
Sa unang aralin ay nagawa nating isalin ang mga ordinaryong parirala at pangungusap sa matematika. Magagamit na natin ang kaalamang iyon upang malutas ang mga number problems. Tingnan ang mga sumusunod na halimbawa.
HALIMBAWA 1
If one number is three times as large as another number and the smaller number is increased by 19, the result is 6 less than twice the larger number. What is the larger number?
Let x = the smaller number
3x = the larger number
x + 19 = the smaller number increased by 19
result = = (equal sign)
2(3x) – 6 = 6 less than twice the larger number
Set up our equation and solve:
x + 19 = 2(3x) – 6
x + 19 = 6x – 6
5x = 25 (Transposition)
5x/5 = 25/5
x = 5 ==> the smaller number
3x = 3(5) = 15 ==> the larger number
Thus, 15 is the larger number.
Ihalili natin ang ating nakuhang sagot sa ating orihinal na equation upang matiyak na tumpak ang ating sagot.
x + 19 = 2(3x) – 6
5 + 19 ≟ 2(15) - 6
24 ≟ 30 - 6
24 = 24
Dahil balanse ang ating nakuhang mathematical expressions, nangangahulugan ito na tumpak ang ating nakuhang sagot.
HALIMBAWA 2
Forty pesos less than 1/2 of Tim’s weekly salary is ₱800. How much does Tim earn each week?
Let x = Tim’s weekly salary
1/2x - 40 = Forty pesos less than ½ of Tim’s weekly salary
is 800 = = 800
Set up the equation and solve:
1/2x – 40 = 800
1/2x = 840 (Transposition)
2(1/2x = 840)
x = 1680
Thus, Tim earns ₱1,680 per week.
Ihalili natin ang ating nakuhang sagot na 1,680 bilang value ng x sa ating
orihinal na equation upang matiyak na tumpak ito.
1/2x – 40 = 800
1/2(1,680) - 40 ≟ 800
840 - 40 ≟ 800
800 = 800
Dahil balanse ang ating nakuhang mathematical expressions, nangangahulugan ito na tumpak ang ating nakuhang sagot.
Ngayon, suriin ang isang problema na kinasasangkutan ng mga geometric figure.
HALIMBAWA 3
If the length of a rectangle is 5 m less than twice the width, and the perimeter is 44 m long, find its length and width.
Sa pagsagot ng ganitong klaseng problema, dapat ay alam natin ang hugis ng mga geometric figures at paano kukunin ang kanilang mga bahagi.
Dahil kinapapalooban ng isang rectangle ang ating problema sa itaas, alalahanin natin ang pigura ng isang rectangle.
Base sa larawan sa itaas, ang isang rectangle ay may apat na gilid. Dalawa ang mahabang sukat o tinatawag na length. Dalawa rin ang maikling gilid na tinatawag namang width. Ang mga length ay may parehong haba o sukat, gayundin ang mga width.
Dapat din nating alamin ang konsepto ng perimeter para masagot natin ang problema.
Ang perimeter ay ang kabuuang mga gilid ng isang rectangle. Ito ay may pormulang:
Perimeter (P) = length + length + width + width or
P = 2 length + 2 width
Dahil batid na natin ang hugis, mga bahagi, at konsepto ng perimeter at rectangle, handa na nating sagutin ang ating word problem.
Let x = width of the rectangle
2x – 5 = length of the rectangle
44 = Perimeter of the rectangle
Gamit ang pormula ng perimeter na:
P = 2 length + 2 width, ihalili natin ang ating mga expressions.
P = 2 length + 2 width
44 = 2(2x – 5) + 2x
44 = 4x – 10 + 2x
6x – 10 = 44 (Transposition)
6x = 54
6x/6 = 54/6
x = 9 m ==> width of the rectangle
2x – 5 ==> 2(9) – 5 ==> 18 – 5 =
13 m ==> length of the rectangle
Thus, the length of our rectangle is 13 m and its width is 9 m.
Ihalili natin ang ating nakuhang mga sagot sa pormula ng perimeter upang matiyak na tama ito.
P = 2 length + 2 width
44 ≟ 2(13) + 2 (9)
44 ≟ 26 + 18
44 = 44
Dahil tama ang nakuha nating equality, tumpak ang nakuha nating sukat ng length na 13 m at width na 9 m.
HALIMBAWA 4
The first side of a triangle is 5 cm less than its second side, the third side is 3 cm more than the first and the perimeter of the triangle is 17 cm long. How long is each side?
` Let x = length of the 2nd side
x – 5 = length of the 1st side
(x – 5) + 3 = length of the 3rd side
17 = perimeter of the triangle
Set up the equation and solve:
side 1 + side 2 + side 3 = Perimeter of a triangle
(x - 5) + x + [(x - 5) + 3] = 17
3x – 7 = 17 (Simplification)
3x = 24 (Transposition)
3x/x = 24/3
x = 8 cm ==> length of the 2nd side
x – 5 ==> 8 – 5 = 3 cm ==> length of the 1st side
(x - 5) + 3 ==> 8 – 5 + 3 = 6 cm ==> length of the 3rd side
Thus, the sides of our triangle measure 8, 3, and 6 cm.
Ihalili natin ang ating mga sagot sa pormula ng perimeter upang matiyak na tama ang mga ito.
side 1 + side 2 + side 3 = Perimeter of a triangle
8 + 3 + 6 ≟ 17
17 = 17
Maaari rin nating ihalili ang 8 na value ng x sa ating original na equation.
(x - 5) + x + [(x - 5) + 3] = 17
(8 - 5) + 8 + [(8 - 5) + 3] ≟ 17
3 + 8 + (3 + 3) ≟ 17
3 + 8 + 6 ≟ 17
17 = 17
Dahil tama ang nakuha nating equality, tumpak ang nakuha nating sukat ng mga sides ng triangle na 3, 8, at 6 cm.
Work Problems
Sa paglutas ng mga work problems, tandaan na kung ang isang tao ay makakagawa ng isang trabaho sa loob ng 5 araw, nakukumpleto niya ang 1/5 ng trabaho sa isang araw, 2/5 sa dalawang araw, at x/5 sa x na araw. Nakakatulong ang isang diagram na ipakita ang relasyong ito.
Complete Job
1/5 ng trabaho ang matatapos sa isang araw.
Sa pangkalahatan, kung aabutin ng b araw upang makumpleto ang isang trabaho, ang bahagi ng trabaho na maaaring gawin sa a na mga araw ay kinakatawan ng fraction na 𝒂/𝒃.
HALIMBAWA 5
Alfred can mow the lawn in 40 minutes and Abel can mow the lawn in 60 minutes. How long will it take for them to mow the lawn together?
Let x = the number of minutes it will take the two men to complete the job together
𝑥/40 = the part of the job that Alfred can do in x minutes
𝑥/60 = the part of the job that Abel can do in x minutes
The relationship used in setting up the equation is:
Part of job done by Alfred + Part of job done by Abel = Complete job.
That is: 𝑥/40 + 𝑥/60=1
Multiply the equation with the LCM of 40 and 60 which is 120.
120(𝑥/40 + 𝑥/60 = 1)
120𝑥/40 + 120𝑥/60 = 120
3x + 2x = 120
5x = 120
5x/5 = 120/5
x = 24 minutes
Thus, the time taken for both of them to mow the lawn together is 24 minutes.
Ihalili natin ang 24 minutes bilang value ng x sa ating orihinal na equation upang matiyak na tumpak ang ating sagot.
𝑥/40 + 𝑥/60 = 1
24/40 + 24/60 ≟ 1
120(24/40 + 24/60 ≟ 1)
3(24) + 2(24) ≟ 120
72 + 48 ≟ 120
120 = 120
Since we got equality, thus, our answer of 24 minutes is correct.
HALIMBAWA 6
A swimming club manager needs to fill the pool in 8 hours and she knows that the built-in water line will take 12 hours to fill the pool. How many hours would it take the auxiliary hose to fill the pool with water?
Let x = number of hours would it take the auxiliary hose to fill the pool completely
8/𝑥 = part of the auxiliary hose to fill the pool in 8 hours
8/12 = part of the built-in water line to fill the pool in 8 hours
Set up the equation and solve.
8/𝑥 + 8/12 = 1
12x(8/𝑥 + 8/12 = 1)
96x/𝑥 + 96𝑥/12 = 12𝑥
96 + 8x = 12x
96 – 96 + 8x – 12x = 12x – 12 – 96 (Transposition)
-4x = -96
-4x/-4 = -96/-4
x = 24 hours
Thus, the auxiliary hose needs a minimum rate of 24 hours to fill the pool.
Substitute 24 as the value of x in the original equations to check if our answer is correct.
8/𝑥 + 8/12 = 1
8/24 + 8/12 ≟ 1
24(8/24 + 8/12 ≟ 1)
192/24 + 192/12 = 24
8 + 16 = 24
24 = 24
Since we got equality, our answer of 24 hours is correct.
Solve the following word problems and check your answers:
1. One number exceeds another number by 5. If the sum of the two numbers is 39, find the smaller number.
2. The denominator of a fraction exceeds the numerator by 4. If 6 is added to the numerator and 2 is subtracted from the denominator, the resulting fraction equals 5. Find the original fraction.
3. A rectangle is 4 times as long as it is wide. If the length is increased by 4 inches and the width is decreased by 1 inch, the area will be 60 square inches. What were the dimensions of the original rectangle?
4. It takes Marie 10 hours to pick sixty bushels of apples. Kyle can pick the same amount in 12 hours. How long will it take if they work together? Round your answer to the nearest hundredths.
ANSWERS: